Recognize that iff(x) is positive for one value in an interval, then f(x) is positive for all values. How do i find increasing & decreasing intervals with differential calculus? Web sign charts are used to analyze functions or solve inequalities. Web sign chart is used to solve inequalities relating to polynomials, which can be factorized into linear binomials. Begin by finding all special values of the polynomial.
Web please look at my chart and tell me if i have it set up correctly. Web signs and sign charts the other method is to use a sign chart with the signs of the factors. And our goal is to figure out which function is which. Select a value of x from each interval and compute f(x). Use first derivative test and the results of step 2 2 to determine whether f f has a local maximum, a local minimum, or neither at each of the critical points.
Increasing & decreasing intervals review. This method is based on the following: 1 a linear factor, ax + b, will be zero at one point (x = b a) and will be positive on one side of the zero and negative on the other. Web please look at my chart and tell me if i have it set up correctly. Find critical points get 3 of 4 questions to level up!
What is a sign chart in calculus?
How to Understand Sign Diagrams
Sign chart calculus qustmama
How to Understand Sign Diagrams
How to Understand Sign Diagrams
How to Understand Sign Diagrams
How to Understand Sign Diagrams
How to Understand Sign Diagrams
Solving Inequalities Using sign chart Calculus 1 YouTube
Sign Chart Math A Visual Reference of Charts Chart Master
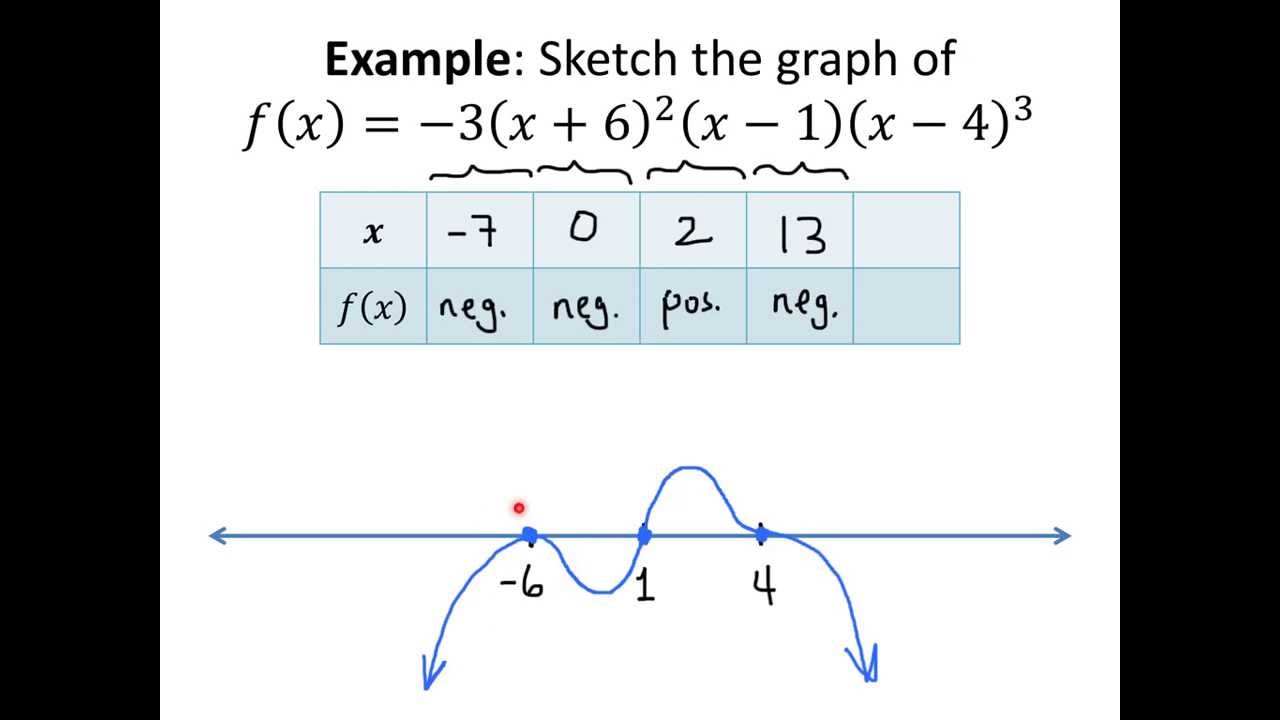
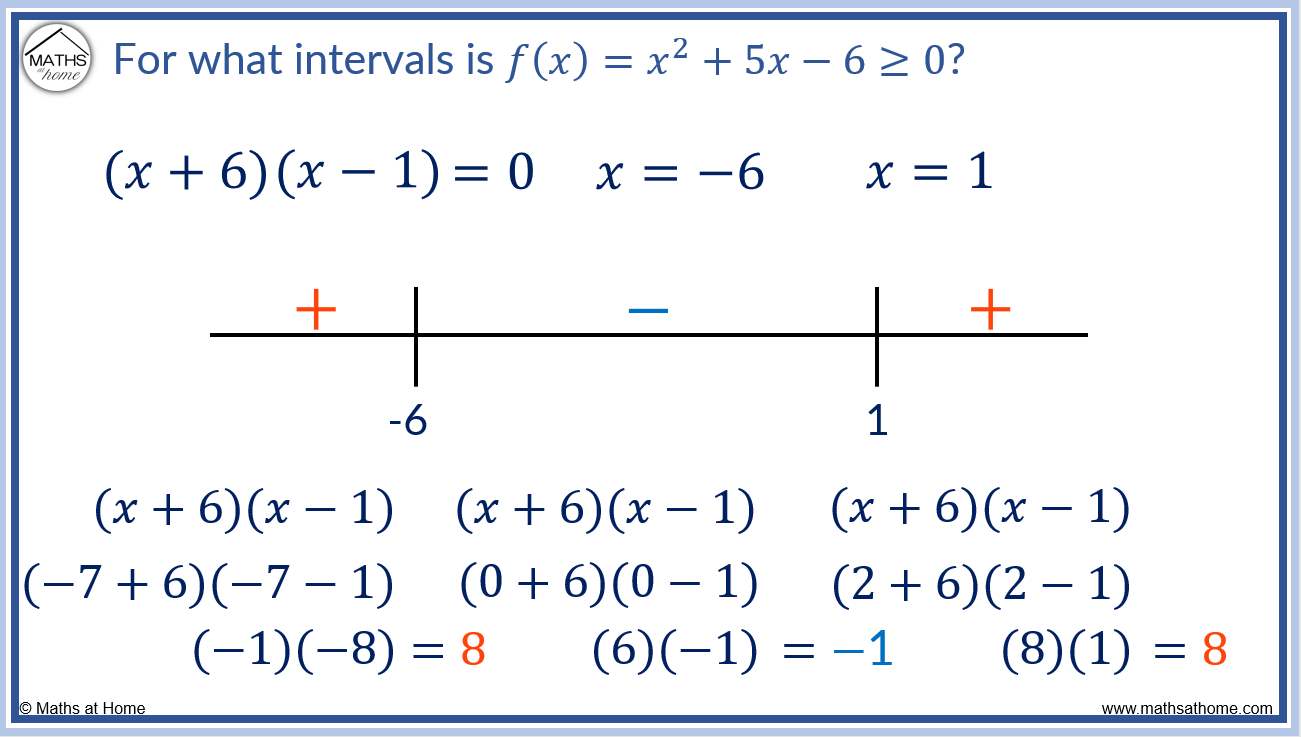
How do i find increasing & decreasing intervals with differential calculus? Web this is an example of how to use sign charts in precalculus and calculus to help locate critical points and graph behavior. For example, of the type (ax+b) (gx+h) (px+q) (sx+t)>0 it could also be less than or less than or equal or greater than or. All the signs should be positive, since the square of a nonzero real number is positive. Web sign chart is used to solve inequalities relating to polynomials, which can be factorized into linear binomials. The intervals where a function is increasing (or decreasing) correspond to the intervals where its derivative is positive (or negative). Select a value of x from each interval and compute f(x). You can ignore the 1/12, since it is a positive constant. Learn how to draw a sign chart here. The f(𝑥) sign diagram displays where the function outputs are positive or negative. Web a comprehensive collection of the most notable symbols in calculus and analysis, categorized by topic and function into charts and tables along each symbol's meaning and example. Web how to create a sign chart to determine where a function is positive and negative. Find critical points get 3 of 4 questions to level up! + + = + + + = + + = + = + = + = = + = + It could also be less than or less than or equal or greater than or equal, but the process is not much effected.
Finding Decreasing Interval Given The Function.
Complete documentation and usage examples. Use first derivative test and the results of step 2 2 to determine whether f f has a local maximum, a local minimum, or neither at each of the critical points. Web use the sign analysis to determine whether f f is increasing or decreasing over that interval. The f(𝑥) sign diagram displays where the function outputs are positive or negative.
It Could Also Be Less Than Or Less Than Or Equal Or Greater Than Or Equal, But The Process Is Not Much Effected.
+ + = + + + = + + = + = + = + = = + = + And our goal is to figure out which function is which. Web graph functions, plot points, visualize algebraic equations, add sliders, animate graphs, and more. The intervals where a function is increasing (or decreasing) correspond to the intervals where its derivative is positive (or negative).
Get A Grid Of Sign Charts For A Function And Its First And Second Derivatives.
Download an example notebook or open in the cloud. Web they provide a concise way to understand the sign of a function within specific intervals. To establish a sign chart (number lines) for f ' , first set f ' equal to zero and then solve for x. Web sign charts are used to analyze functions or solve inequalities.
You Can Ignore The 1/12, Since It Is A Positive Constant.
By examining the intervals where the function is positive, negative, or zero, sign charts aid in identifying critical points, determining the behavior of. 1 a linear factor, ax + b, will be zero at one point (x = b a) and will be positive on one side of the zero and negative on the other. For example, of the type. Web here are instruction for establishing sign charts (number line) for the first and second derivatives.